Researchers in the Numerical Analysis group work on various theoretical issues for applications ranging from signal processing, inverse problems and more specifically computed tomography. This process uses large-scale high-resolution modeling of ocean circulation to models of flow and transport in porous media, and models for wave propagation in liner and nonlinear materials at multiple spatial and temporal scales. Faculty in this group are interested in both the theoretical and implementation issues. The former include development of algorithms, error and stability analysis; the latter concern high-performance computing techniques such as parallel algorithms.
Numerical Analysis
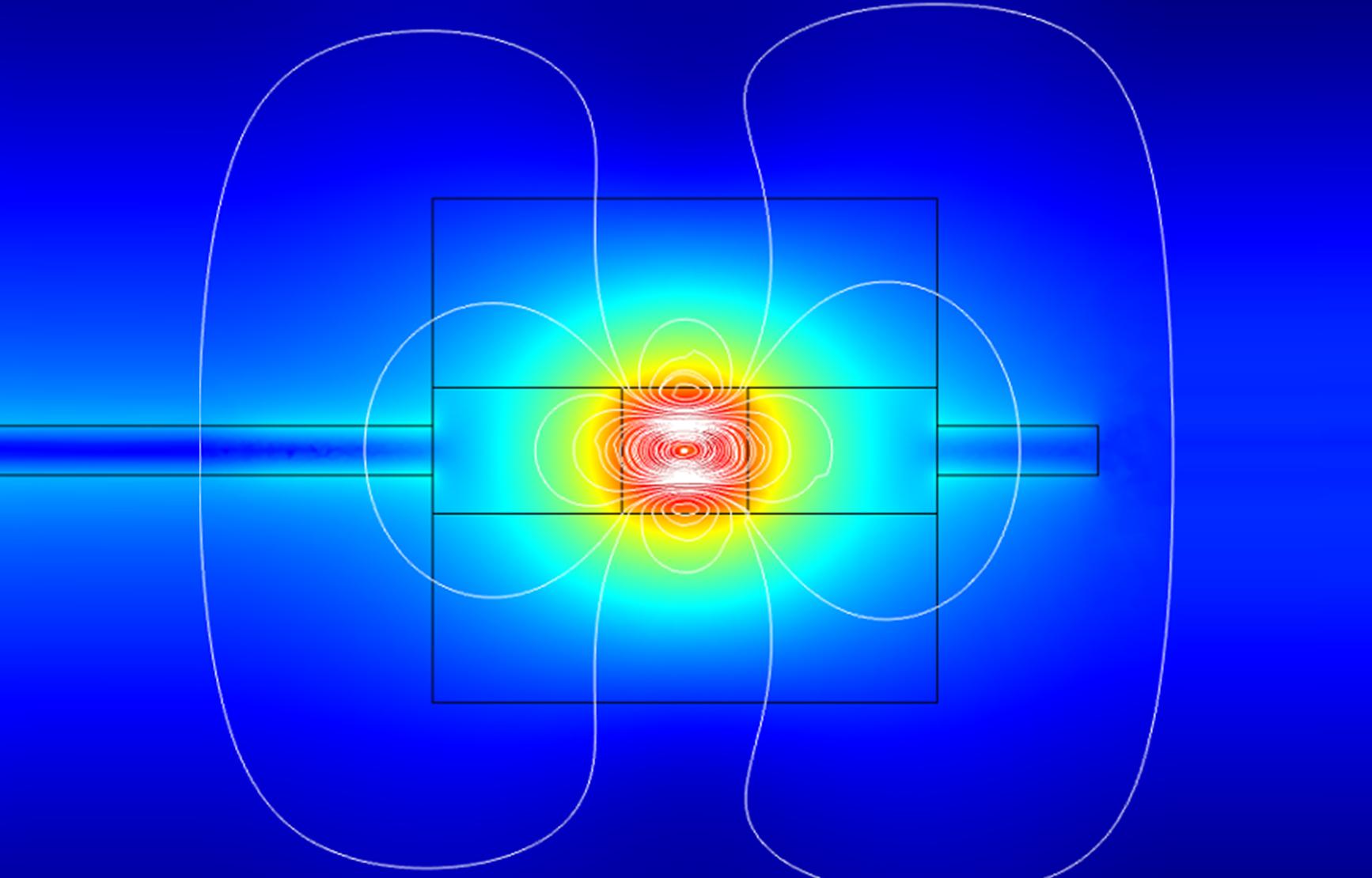
This illustration by Duncan McGregor (Ph.D. '16) depicts induced magnetic field lines within a segment of a segmented Faraday type magnetohydrodynamic power generator. Work by Vrushali Bokil and Nathan Gibson to develop novel numerical discretizations and computational multiscale electromagnetic models incorporating uncertainties in nonlinear material properties will help optimize strategies in the design of advanced power systems as well as electromagnetic nanocomposites.















